Mulyati , Dwi (2003) Penyelesaian permasalahan syarat batas dan nilai eigen pada persamaan diferensial orde II dengan menggunakan algoritma numerov. Undergraduate thesis, FMIPA Undip.
| PDF Restricted to Repository staff only 3112Kb | ||
| PDF 21Kb | |
| PDF 347Kb | |
| PDF 535Kb | |
| PDF 397Kb | |
| PDF 558Kb | |
| PDF 645Kb | |
| PDF Restricted to Repository staff only 484Kb | ||
| PDF 347Kb | |
| PDF 329Kb | |
| PDF 1997Kb |
Abstract
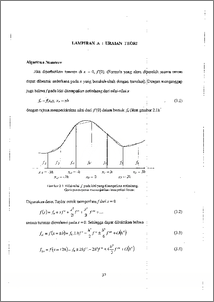
Telah dibuat program untuk menyelesaikan permasalahan syarat batas dan nilai eigen dan Persamaan Diferensial Orde II yang mempunyai bentuk persamaan 2Y k2(xlv = S(x) . Ada dua macaw kasus utama yaitu kasus k2(x) = 0 untuk dx2 potensial elektrostatik pada sebuah muatan bola dan kasus S(x) = 0 untuk aliran papas pada sebuah batang logam atau plat dan partikel bebas dalam .sebuah kotak sate dimensi. Program ini menggunakan Algoritma Numerov dan bahasa pemrograman Delphi 6.0. Algoritma tersebut merupakan metode beda hingga yang merupakan penjabaran dari Deret Taylor. Untuk kasus k2(x) = 0 akan diperoleh grafik hasil komputasi potensial elektrostatik sebagai fungsi jarak dari pusat sebuah muatan bola yang berbentuk eksponensial dan ralat dapat diperkecil dengan suatu angka koreksi. Sedangkan untuk kasus-kasus S(x) = 0 akan diperoleh grafik yang berhimpit untuk fungsi eigen analitik dan numerik. The program for finishing the boundary value and eigenvalue problems from second order differential equation which the equation's style is d2y + k2(.4 = 8(x) cbc2 has been realized. There are two main cases i.e. k2(x) = 0 case for the electrostatic potential in a spherical charge and S(x) = 0 cases for the heat flow in a bar or slab and the free particle in a box for one dimension. This program use Numerov algorithm and Delphi 6.0 program language. The algorithm is finite difference method that is the conversion from Taylor series. For k2(x) = 0 case will be got the computation result graph of the electrostatic potential in a spherical charge which the style is exponential and the error will be becoming small by a correction number. While for S(x) = 0 cases will be got the coincide graph for analytic and numeric eigenfunction.
| Item Type: | Thesis (Undergraduate) |
|---|---|
| Subjects: | Q Science > QC Physics |
| Divisions: | Faculty of Science and Mathematics > Department of Physics |
| ID Code: | 30299 |
| Deposited By: | Mr UPT Perpus 1 |
| Deposited On: | 26 Oct 2011 09:27 |
| Last Modified: | 26 Oct 2011 09:27 |
Repository Staff Only: item control page