Ekawati , Nur (2000) Metode komponen utama pada regresi linier berganda. Undergraduate thesis, FMIPA UNDIP.
| PDF Restricted to Repository staff only 2511Kb | ||
| PDF 18Kb | |
| PDF 232Kb | |
| PDF 359Kb | |
| PDF 265Kb | |
| PDF Restricted to Repository staff only 1088Kb | ||
| PDF 229Kb | |
| PDF 226Kb | |
| PDF 402Kb |
Abstract
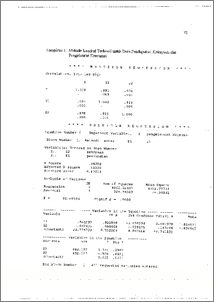
Metode Komponen Utama mangatasi multikolinieritas dengan edi a menghapus pengaruh yang menyebabkan masalah multikolinieritas terjadi yaitu dengan menggunakan hanya sebagian dan selurnh himpiman komponen utama di dalarn model. Dengan kata lain, penaksir komponen utama untuk yaitu 13p, diperoleh dengan Cara memperlakukannya sebagai penaksir kuadrat terkecil terkendala yaitu dengan merninimumkan jnrnlah kuadrat residual terhadap kendala 8e T 0 dimataa 32 adalah vektor koefisien regresi komponen utama yang bersesuaian dengan komponen utama yang dihapus dari model. Jika kendala tersebut benar, penaksir I3 Pc akan mernpakan penaksir yang tak bias dan A merupakan penaksir yang "lebih bail: " dari penaksir kuadrat terkecil 13. dilihat dari varian dan rata-rata kuadrat residualnya. Principal component method solves the problem of multicollineatity by removing the affect which cause it, that is by using less than the full set of principal components in the model. In other way, principal component estimator for p, that is Ppc , is obtained by using it as the restricted least squares estimator, that is to minimize sum square error subject to 82 = 0, where 82 is the vector of principal component regyession coefficients associated with principal components removed fi•om the model. If the restrictions are correct, the estimator pPc will be unbiased A and it's "better" than the least squares estimator f3, which is viewed from it variance and mean square error. changing the content, translate the submission to any medium or format for the purpose of preservation. The author(s) or copyright owner(s) Also agree that LINDIP-iR may keep. more than one.copy of. this submission for purpose of security, backup and. preservaton: ( http://eprints.0 diX. a c.id )
| Item Type: | Thesis (Undergraduate) |
|---|---|
| Subjects: | Q Science > QA Mathematics |
| Divisions: | Faculty of Science and Mathematics > Department of Mathematics |
| ID Code: | 31750 |
| Deposited By: | Mr UPT Perpus 1 |
| Deposited On: | 24 Nov 2011 14:02 |
| Last Modified: | 24 Nov 2011 14:02 |
Repository Staff Only: item control page